Quadrilateral
A plane figure bounded by four-line segment is called the quadrilateral. The area enclosed by the four sides must not be zero.
A simple quadrilateral has four sides and four angles.
Sum of the angle of the quadrilateral is always 360°
∠A+∠B+∠C+∠D=360°
Area of quadrilateral ABCD = 1/2[AC(DE+BF)]
DE and BF are the perpendiculars on diagonal AC.
Types of Quadrilateral
Parallelogram:
- A quadrilateral whose opposite sides are equal and parallel
- Opposite sides angle are equal(∠A=∠C)and (∠B=∠D).
- Sum of any adjacent angle is 180°
- Diagonals bisect each other.
- Diagonals need not be perpendicular or equal.
- Each diagonals divides a parallelogram into two triangle ofequal area.
- Area of a parallelogram is double the area of the triangle formed by diagonals.
- Bisectors of the angle of a parallelogram form a rectangle.
- A parallelogram inscribed inside a circle is rectangle.
- A parallelogram circumscribed about a circle is a rhombus.
- AC2+BD2=AB2+BC2+CD2+AD2=2(AB2+BC2)
- Area of parallelogram ABCD= Base *Height
- A parallelogram is a rectangle if its diagonal are equal.
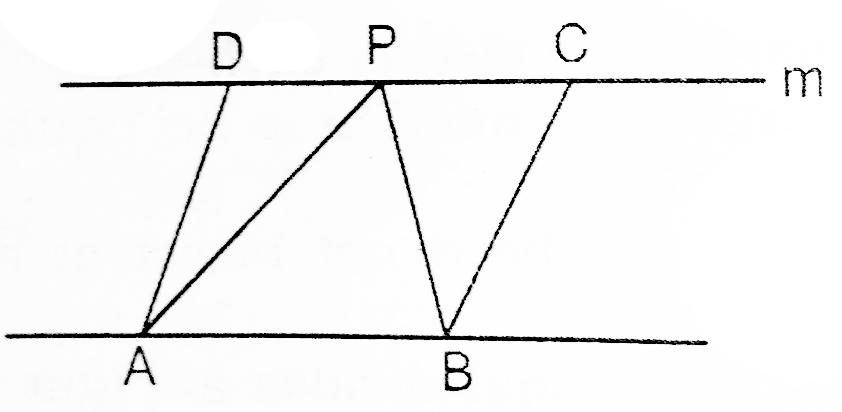
- Parallelogram that lies on the same base and between the same parallel lines are equal in area.
area of Parallelogram ABCD=Area of Parallelogram ABPQ
- If a triangle and a parallelogram are on the same base and between the same parallel lines, the area of triangle is half of the parallelogram.
Area of triangle APB=1/2(area of parallelogram ABCD)
Rectangles:
- A rectangle is a parallelogram whose all angles 90°.
- Diagonals are equal and bisect each other but not necessarily at 90°.
- A square has maximum area for a given perimeter of rectangles.
- Figures formed by joining the midpoints of the adjacent side of the rectangle is a rhombus.
- Angle Bisectors of a rectangle form another rectangle.
- Area of rectangle=length*breadth
- Diagonals of the rectnagle[AC=BD=√(l2+b2)]
Square:
- A square is a rectangle with all its side equal and all the angle equal to 90°.
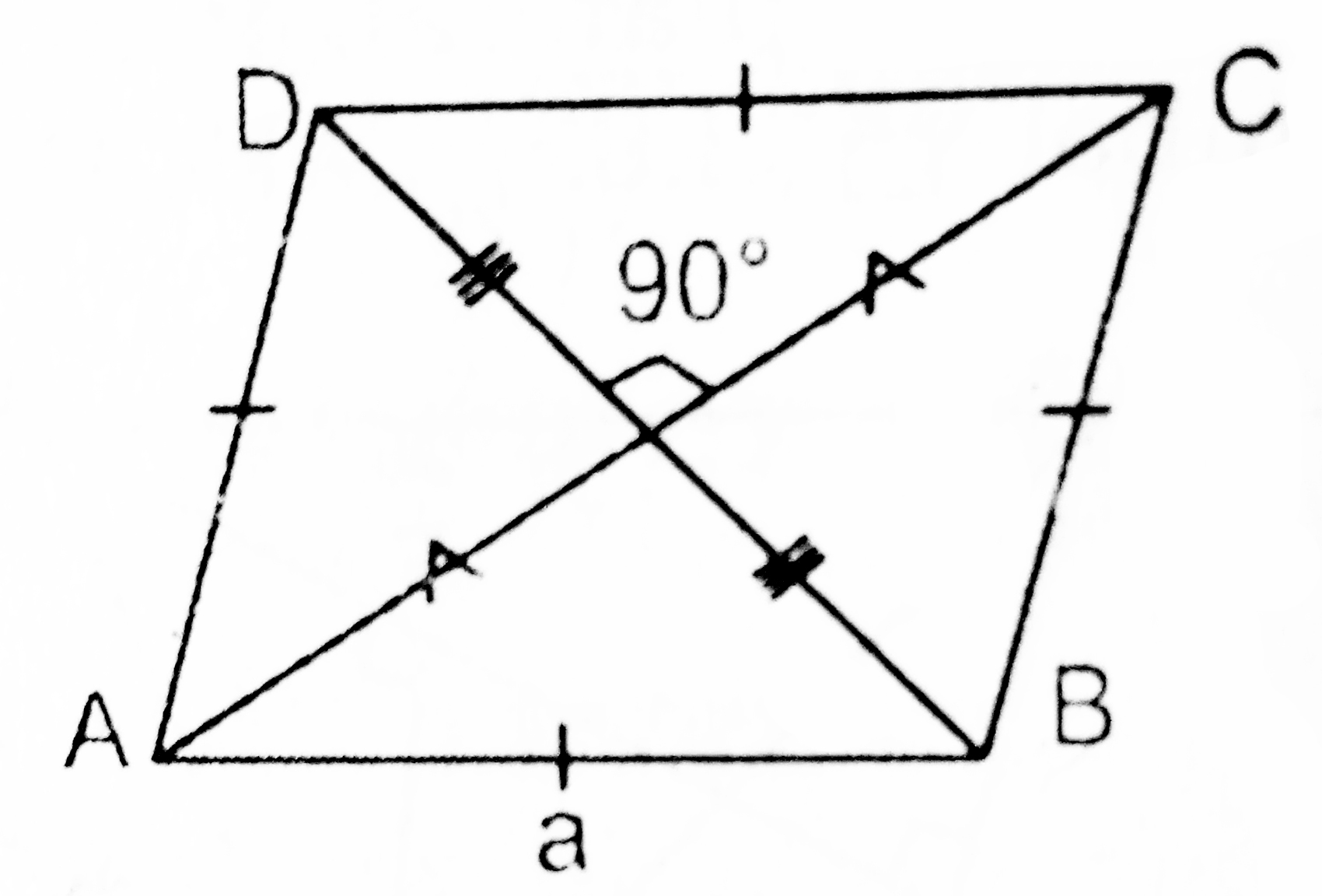
- Diagonals are equal and bisect each other at 90°
- Figure formed by joining the midpoint of the sides of square is a square.
- Area= a2
- Diagonal=√2*a
Rhombus:
- A parallelogram having all its sides equal is a rhombus.
- Diagonals of the rhombus bisect each other at a right angle but they are not necessarily equal.
- A rhombus may or may not be a square but all the square are rhombus.
- The figure formed by joining the midpoint of the adjacent sides of a rhombus is a rectangle.
- A parallelogram is a rhombus if its diagonals are perpendicular to each other.
- Area of rhombus=1/2(product of diagonal)
- here AC and BD are diagonal.
- AC2+BD2=4AB2
Trapezium:
- A quadrilateral is a trapezium with only two sides parallel to each other.
- The sum of co-interior angles are equal to 180(∠A+∠D=180°, B +C=180)
- Area of trapezium =1/2[(sum of parallel sides)*heights]
= 1/2[(AB+CD)*DM]
- sum of the square of diagonal =(sum of square of non-parallel sides) +2(products of parallel sides)
AC2+BD2=BC2+AD2+2AB*CD
- If E and F are the midpoints of two non-parallel sides AD and BC respectively then
EF=1/2(AB+DC) 
- AO*OD=OC*BO
- E and F are the mid-points of the side AB and AC respectively then,
EF=1/2(BC) and EF parllel BC
Polygon:
- A closed figure bounded by three or more than three straight lines.
Convex Polygon:
- A polygon in which none of its interior angle is more than 180°.
Concave Polygon:
- A polygon in which at least one of its interior angle is more than 180°
Regular Polygon:
- A polygon in which all the sides are equal and also the interior angles are equal is called a regular polygon.
- if n is the total no sides of the polygon then
- Sum of its interior angle is (n-2)*180°
- Each exterior angle=360°/n
- Sum of all exterior angle is 360°
- Sum of an interior angle and exterior angle is 180°
- Number of diagonals =n(n-3)/2
More from us:
BYJU'S Exam Prep Presents Test Series to your dream job. Get Unlimited Access to all Mock Tests on a single card. Hurry up!! Get your Test Series now.
SSC & Railways Test Series: Unlimited Access to all the Mocks | Register Now
Are you appearing for SSC CGL Tier-II 2019-20 Exam? If yes then we have a dedicated course for you in which we will prepare you not only for the upcoming SSC CGL Tier II 2019 exam but also for the SSC CGL Tier III 2019-20 exam. So, don’t lose the opportunity to grab your govt job with the help of BYJU'S Exam Prep. To start a free trial follow the link given below.
SSC CGL Tier-II: A Comprehensive Course Hindi (Batch 3)
SSC CGL Tier-II: A Comprehensive Course (English Medium) (Batch - 2)
Attempt subject wise questions in our new practise section' Click here
Download Gradeup, the best government exam app for Preparation
BYJU'S Exam Prep today to score better!













Comments
write a comment