Boundary Layer – Definition, Types & Characteristics

What is a Boundary Layer in Boundary Layer Theory?

The boundary layer in boundary layer theory is a thin layer of fluid formed over the solid surface or internal flow through pipes due to velocity or temperature gradient. Boundary layer theory is also used to study the internal flow of fluids. The boundary layer is basically classified as

• Hydrodynamic Boundary Layer
• Thermal Boundary Layer

Hydrodynamic Boundary Layer for External Flow

When the real fluid flows over the solid body, the fluid is adhered to the solid boundary and assumes no-slip condition. i.e., the velocity of the fluid particle which is adhered to the boundary becomes equal to the velocity of the boundary. If the boundary is stationary, the velocity of fluid particles which are adhered to the stationary boundary becomes zero. Boundary layer theory is to determine the loss of energy for fluid flow in channels. The velocity gradients will be developed perpendicular to the solid boundary. The velocity profile for the real fluid flow over the horizontal solid surface is shown below.

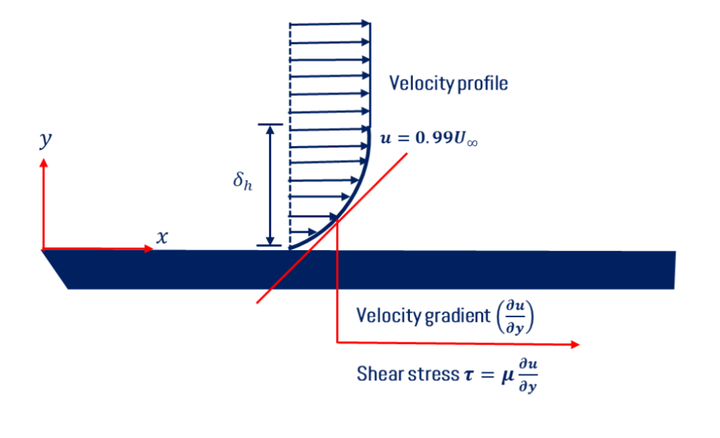
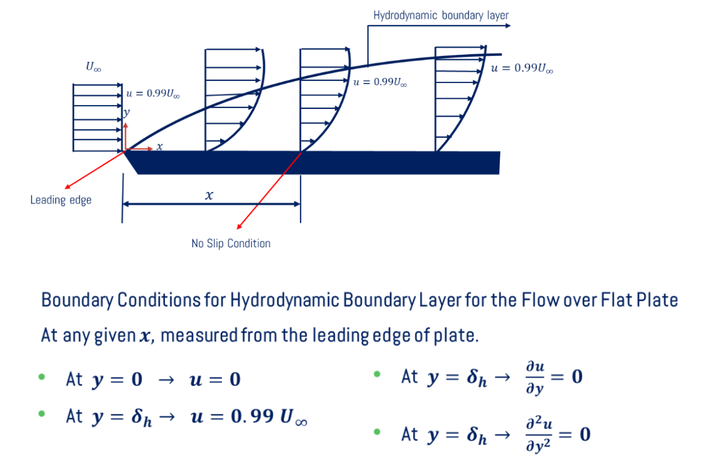
• Hydrodynamic boundary layer thickness (δ_h) is defined as the distance measured perpendicular to the boundary up to which the fluid velocity becomes 99% of free stream velocity (U_∞). To get a hydrodynamic boundary layer, first draw velocity profiles at every point on the solid surface and mark the point perpendicular to the boundary where the fluid has 0.99U_∞. The locus of all these points is termed as Hydrodynamic boundary layer. The formation of the boundary layer over the horizontal solid surface/flat plate is shown in the figure below.

Hydrodynamic Boundary Layer for Internal Flow

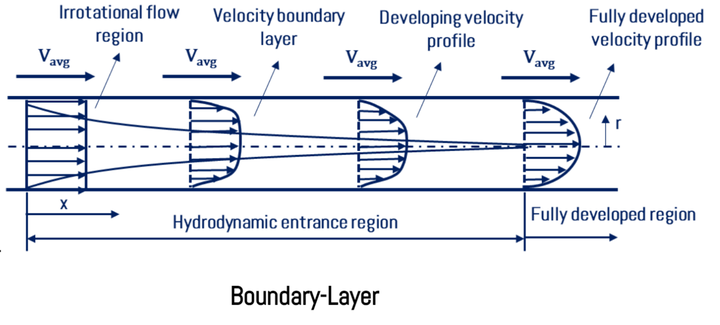
When fluid flows through the circular/non-circular pipe, the pipe’s surface’s velocity is zero, and the maximum velocity (u_max) at the pipe center. The average velocity (u_avg) fluid is used to analyze fluid flow characteristics. The velocity profile for the fluid which is flowing through the pipe is shown below.

Thermal Boundary Layer for External Flow

When the fluid with free stream temperature (T_∞) flows over the hot solid surface with a temperature (T_s). The temperature gradients will be developed perpendicular to the solid boundary. The temperature profile for the real fluid flow over the horizontal solid surface is shown below.

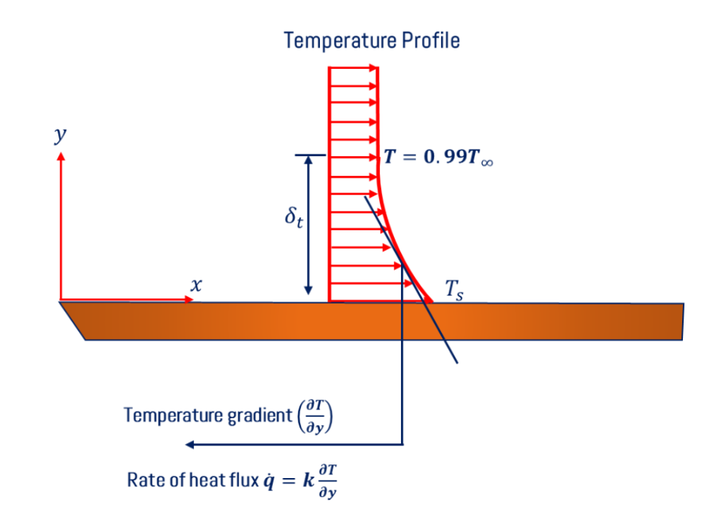
• Thermal boundary layer thickness (δ_t) is the distance measured perpendicular to the boundary up to which the fluid temperature becomes 99% free stream temperature (T_∞). To get the thermal boundary layer, first, draw temperature profiles at every point on the solid surface and mark the point perpendicular to the boundary where the fluid has a temperature of 0.99T_∞. The locus of all these points is termed the thermal boundary layers. The formation of a thermal boundary layer over the horizontal solid surface/flat plate is shown in the figure below.

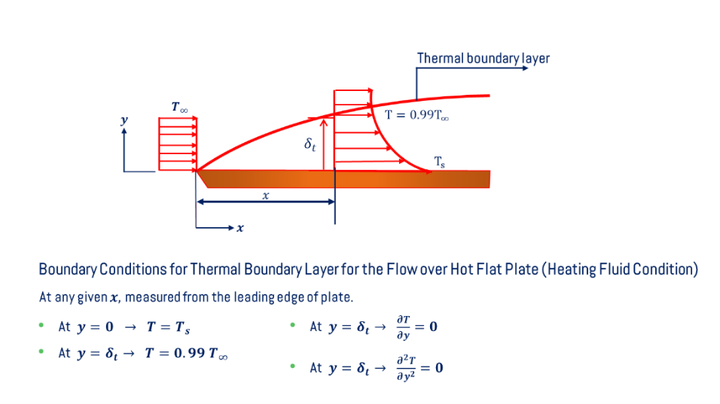
Thermal Boundary Layer for Internal Flow

When fluid flows through the hot circular/non-circular pipe, the temperature at the pipe’s surface temperature of the hot pipe (T_s) and the minimum temperature (T_min) at the pipe center. The average temperature (T_avg) fluid is used to analyze the thermal characteristics of fluid flow. The temperature profile for the fluid which is flowing through the pipe is shown below.

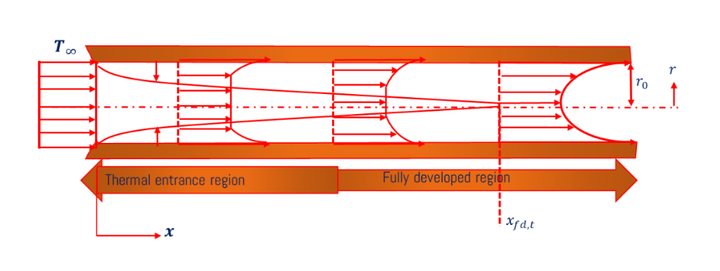
Relationship between Hydrodynamic (δ_h) and Thermal (δ_t) Boundary Layer Thicknesses

L.Prandtl proposed the relationship betweenδ_hand δ_tafter analyzing different hydrodynamic and thermal boundary layer formations in different cases. Prandtl number is a dimensionless number, and it is defined as the ratio of momentum diffusivity(ν)and thermal diffusivity(α). The relationship between hydrodynamic and thermal boundary layer thicknesses is given below.

δ_h/δ_t=Pr^1/3

Here,

• δ_h= Hydrodynamic boundary layer thickness
• δ_t= Thermal boundary layer thickness
• Pr= Prandtl number =v/α

Results of Hydrodynamic and Thermal Boundary Layer for Laminar External Fluid Flow

For the laminar hydrodynamic boundary layer, first, the velocity profile is derived by using boundary conditions, the boundary layer thickness (δ_h), wall and average shear stress, and local and average drag coefficient by using Von Karman and Blasius equations. When the fluid flows over the horizontal flat solid surface, the results for laminar flow in the case of both hydrodynamic and thermal boundary layers are given in the table below.

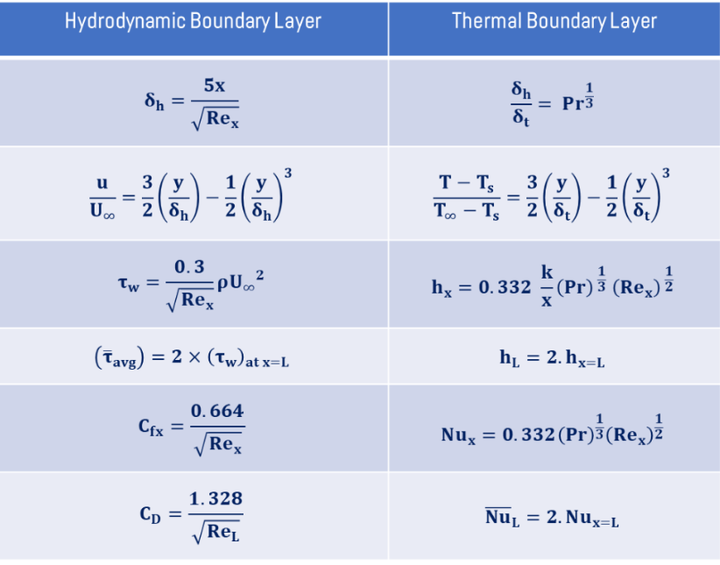
Here,

• δ_h= Hydrodynamic boundary layer thickness
• δ_t= Thermal boundary layer thickness
• u= Velocity of fluid flow
• T= temperature of fluid flow.
• τ_w= Wall shear stress
• h_x= Local heat transfer coefficient
• Re_x= Local Reynold’s number
• Pr= Prandtl number
• τ_avg= Average shear stress
• h_L= Average heat transfer coefficient
• C_fx= Local skin friction coefficient
• Nu_x= Local Nusselt number
• C_D= Coefficient of drag
• Nu_L= Average Nusselt number

Important Topics for Gate Exam
Composite Beams	Compression Members
Concurrent Force System	Conditions For Deadlockn Operating System
Conduction Heat Transfer	Consensus Theorem
Constants in C	Convection Heat Transfer
Coplanar Force System	Cut Set Matrix