Shallow Foundation: Types and Uses

What is Shallow Foundation?

A shallow foundation is a foundation type, and foundations are those structures designed to transfer a load of superstructure beneath a layer of soil. The design of the shallow foundation is carried out when the bearing capacity of the top layer of the soil is sufficient enough to transfer the load of the superstructure.

In the case of a shallow foundation, the depth of its foundation is relatively less, and it would be lesser than the width of the foundation. In such cases bearing capacity of soils should be such that it can bear the load of the superstructure and not allow the differential settlement of the foundations. This is a high-scoring concept for the GATE exam. Let’s understand more about the bearing capacity of soils.

Bearing Capacity of Soils

The bearing capacity of soil is the load-carrying capacity of the soil. Based on the bearing capacity of the soil type, a foundation is preferred for the design of structures. The bearing capacity of soils can be classified into many types based on considering different parameters.

Ultimate Bearing Capacity or Gross Bearing Capacity (q_u)

It is the least gross pressure that will cause the shear failure of the supporting soil immediately below the footing.

Net Ultimate Bearing Capacity (q_nu)

The net pressure applied to the footing by external loads will initiate failure in the underlying soil. It is equal to the ultimate bearing capacity minus the stress due to the weight of the footing and any soil or surcharge directly above it. Assuming the density of the footing (concrete) and soil (γ) are close enough to be considered equal, then

q_nu = q_u – γD_f

Where D_f is the depth of footing

Safe Bearing Capacity

It is the bearing capacity after applying the factor of safety (FOS). safe bearing capacity can be classified into two types,

1. Safe net bearing capacity (q_ns)

The net soil pressure that can be safely applied to the soil considering only shear failure.

It can be expressed as q_ns = q_nu/FOS.

2. Safe gross bearing capacity (q_s )

It is the maximum gross pressure that the soil can carry safely without shear failure.

It can be expressed as q_s = q_ns + γD_f.

Allowable Bearing Pressure

The maximum soil pressure without any shear or settlement failure that can be allowed for a particular structural member is called allowable bearing pressure.

Types of Shallow Foundation

Shallow foundations are the foundation used to transfer a load of the superstructure to the subsequent layer of the soil. A shallow foundation is provided when the top layer of the soil has sufficient bearing capacity to transfer the load. The types of shallow foundations depend on various parameters including their shape and size.

Shallow foundations can be classified into the following types:

• Isolated footing
• Wall footing
• Strap footing
• Mat footing
• Combined footing

Method to Determine Bearing Capacity in Shallow Foundation

Determination of the bearing capacity is very important before designing the structure. The bearing capacity of soil can be determined with the help of different methods based on the soil type and foundations.

(i) Rankine’s Method (C – ∅ soil)

With the help of Rankine’s method bearing capacity of the C-∅ soil is determined based on the following equations.

q_u = γD_f tan²{45° + (φ/2)}

(ii) Bells Theory (C – ∅)

q_u = CN_c + γD_fN_q

where N_c and N_q are bearing capacity factors.

(iii) Fellinious Method (Cohesive soil)

• The failure is assumed to occur by slip, and the consequent heaving of a soil mass is on one side.

Q_ult = [W.I_r + CR ]/b.I₀

q_u = 5.5 C

• Location of Critical circle

(iv) Prandtl Method: (C – ∅)

For strip footing

q_u = CN_c + γD_fN_q + 0.5γBN_γ

For C-soil

N_c 5.14, N_q = 1, N_γ = 0

(v) Terzaghi Method (C – ∅)

With this method, an analysis of the bearing capacity of C – ∅ soil is carried out. This analysis is carried out based on the following assumptions:

1. The soil mass below the footing is considered homogenous and isotropic.
2. The shear strength of the soil is calculated with the help of Coulumb’s criteria of failure.

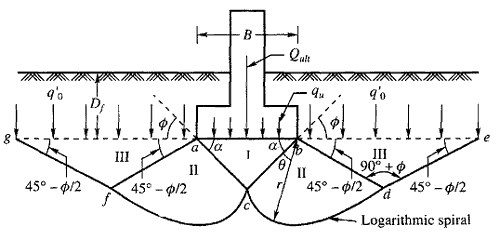
For strip footing

q_u = CN_c + γD_fN_q + 0.5γBN_γ

For square footing

q_u = 1.3CN_c + γD_fN_q + 0.4γBN_γ

For rectangular footing

q_u = {1 + 0.3(B/L)}CN_c + γD_fN_q + 0.5{1 – 0.2(B/L)}γBN_γ

For circular footing

q_u = 1.3CN_c + γD_fN_q + 0.3γBN_γ

where,

• D = Dia of circular footing
• CN_c → Contribution due to the soil’s constant component of shear strength.
• γD_fN_q → Contribution due to surcharge above the footing
• 0.5γBN_γ → Contribution due to bearing capacity due to self-weight of soil.

Bearing capacity factors

N_q = N_φθ^πtanφ
where,

• N_φ= influence factor
• N_φ = tan²{45° + (φ/2)}
• N_γ = 1.8 tan φ(N_q – 1)
• N_C = cot φ(N_q – 1)

For C-soil

N_C = 5.7, N_q = 1, N_γ = 0

(vi) Skemptons Method (c-soil)

This method gives the net ultimate value of bearing capacity.

Applicable for purely cohesive soils only.

q_nu = CN_c

For strip footing.

N_c = 5 to 7.5

For circular and square footing.

N_c = 6 to 9

Values of N_C

(D_f/B) = 0 ⇒ i.e. footing at the surface.
Then N_C = 5 For strip footing.
N_C = 6.0 For square and circular footing.
where D_f = Depth of foundation.

• If 0 ≤ (D_f/B) ≤ 2.5
  N_c = 5[1 + 0.2(D_f/B)] For strip footing
  N_c = 6[1 + 0.2(D_f/B)] For square and circular footing.
  B = D in the case of circular footing.
  N_c = 5[1 + 0.2(B/L)][1 + 0.2(D_f/B)] For rectangular footing
• If (D_f/B) ≤ 2.5 ⇒ N_C =7.5
  For strip footing
  N_C = 9.0 for circular, square and rectangular footing.

(vii) Meyorhoff’s Method → (C – ∅ soil)

q_u = CN_cs_cd_ci_c + γD_fN_qs_qd_qi_q + 0.5γBN_γs_γd_γi_γ

(viii) IS code Method

q_nu = CN_cs_cd_ci_c +γD_f(N_q – 1)s_qd_qi_q + 0.5γBN_γs_γd_γi_γ

Comparison of Shallow Foundation and Deep Foundation

Shallow foundation and deep foundation both are types of foundation. These foundation types are classified based on the depth of the foundation. If the depth of the foundation is lesser than the width of the foundation then it is called a shallow foundation and if the depth of the foundation is greater than the width of the foundation then it is called a deep foundation.

A deep foundation is provided at great depth when the above layer of soil does not have sufficient bearing capacity to transfer the load. While shallow foundations are provided at a shorter depth of foundation and in some cases, they can also be placed at the ground level.

Other Important GATE Topics
Critical Path Method	Zero Force Member In A Truss
Difference Between Compiler and Assembler	Transverse Loading
Geometric Design Of Highways	Moment Area Method
Drainage System	Gear Profiles
Impulse An Momentum	Uniformly Varying Load

If you are preparing for ESE/ GATE or other PSU Exams (Civil Engineering), then avail Online Classroom Program for ESE and GATE CE

Online Classroom Program for ESE/GATE CE

You can avail of BYJU’S Exam Prep Test Series, specially designed for all Civil Engineering Exams

BYJU’S Exam Prep Test Series ESE/GATE CE