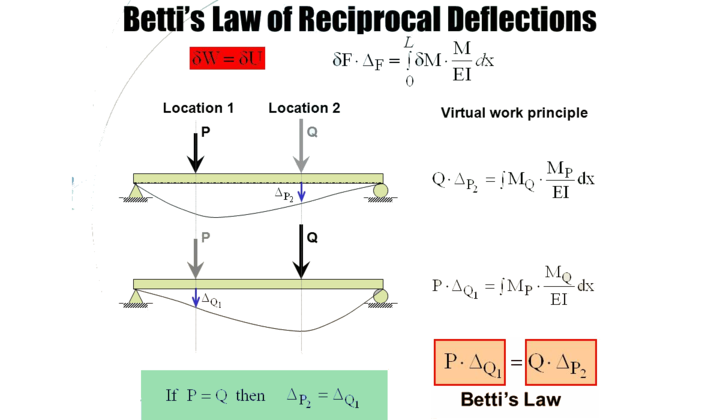
Betti’s Theorem
By BYJU'S Exam Prep
Updated on: September 25th, 2023

Betti’s Theorem is one of the many laws used for structural analysis. In Civil Engineering, for Structural Analysis, the displacement of a member is one of the very important parameters of design. Betti’s theorem is a basic structural analysis law used to calculate the deformation of a member in the structure.
Betti’s theorem is based on the principle of virtual work. In this article, all of Bettis theorem has been discussed in detail along with its application and a few examples.
Download Formulas for GATE Civil Engineering – Engineering Mechanics
Table of content
What is Betti’s Theorem?
Betti’s Theorem is a generalized case of Maxwell’s reciprocal theorem and is widely used for structural analysis.
Betti’s Theorem Statement
According to this theorem, the virtual work done by the P system of forces in going through the deformation of the Q system of forces is equal to the virtual work done by the Q system of forces in going through the deformation of P systems of forces.
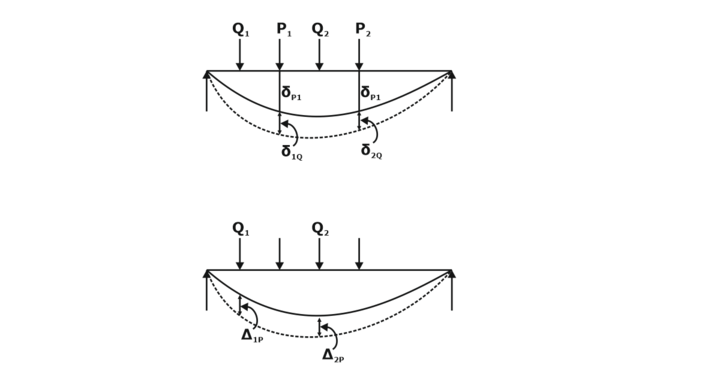
Betti’s Theorem Proof
Consider the following system shown above,
Virtual work done by the P system of forces due to the displacements caused by the Q system of forces = P1 δ1Q + P2 δ2Q
Similarly,
Virtual work done by the Q system of forces due to the displacements caused by the P system of forces = Q1 ∆1P+Q2 ∆2P
So, as per Maxwell-Betti’s Theorem
P1 δ1Q+P2 δ2Q = Q1 ∆1P+Q2 ∆2P
Download Formulas for GATE Civil Engineering – Engineering Mechanics
Betti’s Theorem in Structural Analysis
Bett’s theorem in structural analysis is based on the principle of virtual energy, which says that work done by the internal forces in the structure is the same as that done by the external forces in the structure. With the help of this theorem, deformation at different points in the member can be compared with each other.
Betti’s Theorem PDF
Maxwell-Betti’s law establishes a relation of reciprocal deflection, which states that displacement at a point due to point load at another point is equal to the displacement at another point due to the load at the first point of a member. This theorem is only valid for a stable structure under the elastic limit.
Applications of Betti’s Theorem
Betti’s theorem has many applications in structural analysis. This theorem is used to calculate the deformations of a member at a point in the structure when deformation at another point is known. This theorem can also draw the influence line diagrams and derive the boundary element method. This theorem can also be used in the case of the design of compliant mechanisms by using a topology optimization approach.
Maxwell’s reciprocal theorem is one of the main applications of Betti’s theorem, which has many uses in basic structural analysis. With the help of Maxwell-Betti’s theorem, displacements at two different points of a member can be correlated with the help of the virtual energy principle.
Download Formulas for GATE Civil Engineering – Structural Analysis
Comparison Between Maxwell’s Reciprocal Theorem and Betti’s Theorem
Maxwell’s reciprocal theorem gives a special case known as Betti’s theorem. Both theorems relate the deformation and point loads acting on a member at different points. Here some comparisons between these theorems are listed below, which makes a better understanding of these theorems.
- Betti’s theorem relates the series of point loads with the deformation at other points, while Maxwell’s theorem can only be used for two points.
- Both theorems are based on the work-energy principle, which states that work done by the set of internal forces is the same as that done by the set of external forces.
Examples of Betti’s Theorem
As we discussed earlier, Betti’s theorem has many basic structural engineering applications. And both Maxwell’s and Betti’s laws are used to find deformations but for different loading conditions over the member. Concepts of both theorems can be better understood by solving a few examples. Here one such example is shown below: