Mohr’s Circle for Plane Stress and Plane Strain Notes for Civil Engineering Exams
By BYJU'S Exam Prep
Updated on: September 25th, 2023

IT Kharagpur will conduct GATE 2022 and the official notification for the examination is released now. The application form link for GATE 2022 is now open for candidates. Two new papers ie. Naval Architecture & Marine Engineering and Geomatic Engineering are introduced this year in GATE 2022. Two new subjects Environment Sciences(ES), Humanities and Social Sciences(XH) were added to the GATE 2021 exam. The GATE 2022 exam will be conducted on the 5th,6th,12th and 13th of February 2022.
If a student focuses on cracking GATE 2022, they must check the GATE 2022 syllabus before starting their preparation. Candidates are advised to check the GATE 2022 exam pattern along with the Syllabus of GATE 2022. Read the complete BYJU’S Exam Prep for GATE article to know more about the GATE syllabus 2022.
Mohr’s circle is the locus of points representing the magnitude of normal and shear stress at the various plane in a given stress element. Graphically, a variation of normal stress and shear stress are studied with the help of Mohr’s circle.
IT Kharagpur will conduct GATE 2022 and the official notification for the examination is released now. The application form link for GATE 2022 is now open for candidates. Two new papers ie. Naval Architecture & Marine Engineering and Geomatic Engineering are introduced this year in GATE 2022. Two new subjects Environment Sciences(ES), Humanities and Social Sciences(XH) were added to the GATE 2021 exam. The GATE 2022 exam will be conducted on the 5th,6th,12th and 13th of February 2022..
If a student focuses on cracking GATE 2022, they must check the GATE 2022 syllabus before starting their preparation. Candidates are advised to check the GATE 2022 exam pattern along with the Syllabus of GATE 2022. Read the complete BYJU’S Exam Prep for GATE article to know more about the GATE syllabus 2022.
Mohr’s circle is the locus of points representing the magnitude of normal and shear stress at the various plane in a given stress element. Graphically, a variation of normal stress and shear stress are studied with the help of Mohr’s circle.
σ1 and σ2 are Principal Stresses, then normal and shear stress on the lane which is inclined at angle ‘θ’ from the major principal plane, then


Normal stress
![]()
Shear stress
![]()
The General State of Stress at an Element:
- If σx and σy are normal stress on vertical and horizontal plane respectively and this plane is accompanied by shear stress then normal stress and shear stress on the plane, which is inclined at an angle θ from the plane of
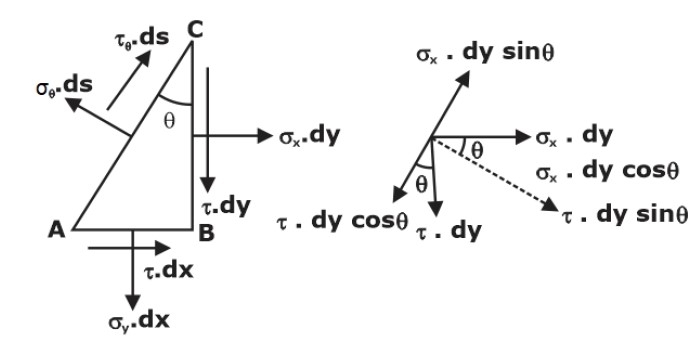
then,
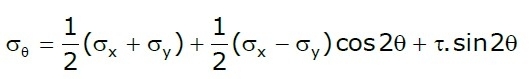
![]()
- Maximum and Minimum Principle Stresses are:
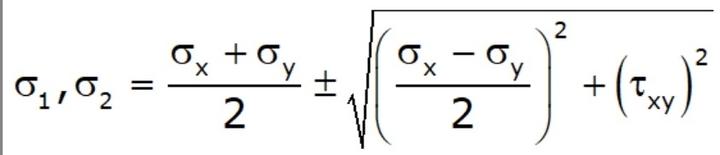
- The radius of Mohr’s circle:
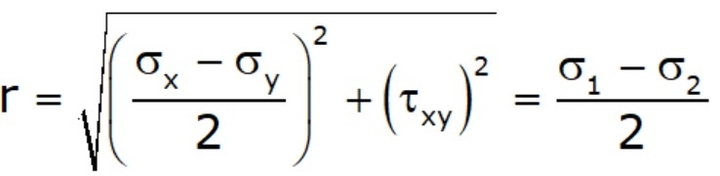
Strength of Materials

Observations from Mohr’s Circle
The following are the observations of Mohr’s circle as
- At point M on circle σn is maximum and shear stress is zero.
∴ Maximum principal stress ≡ coordinate of M
- At point N on the circle, σn is minimum and shear stress τ is zero.
∴ minimum principal stress ≡ coordinate of N
- At point P on Circle τ is maximum.
Maximum shear stress ≡ ordinate of P(i.e. the radius of the circle)
- Also, normal stress on the plane of maximum shear stress:
σn = abscissa of P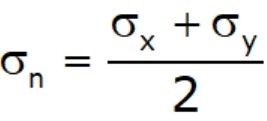
Where σn ≡ Average stress - Mohr’s circle becomes zero at a point if the radius of the circle has the following consideration.
- The radius of the circle:
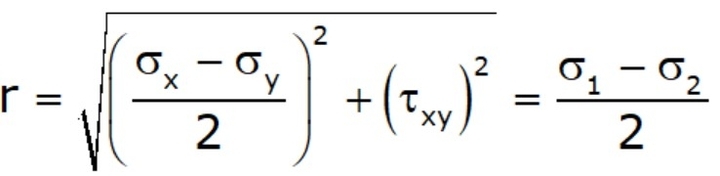
- If σx = σy, then radius of Mohr’s circle is zero and τxy = 0.
- The sum of normal stresses acting on perpendicular faces of a plane stress elements is constant and independent of the angle θ.
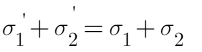
Strain analysis:
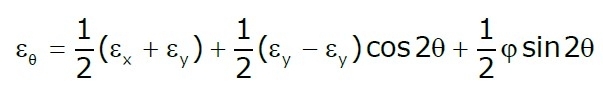
![]()
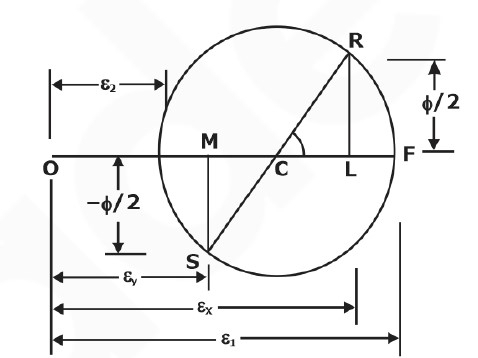
![]()
![]()
Relation between Principle strain and stress:
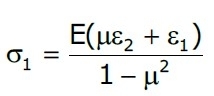

Strain Rosetts:
Rectangular Strain Rosette:
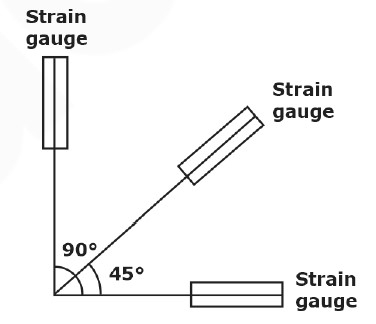
![]()
Equiangular Strain Rosette (delta strain rosette):
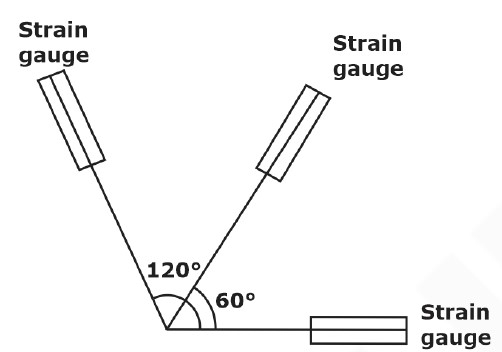
![]()
![]()
![]()
Accelerate your GATE 2022 preparations with Test Series:
Click Here Avail Mechanical Engineering Test Series (160+ Mock Tests)
Click Here Avail Civil Engineering Test Series (180+ Mock Tests)
Click Here Avail Computer Science Engineering Test Series (112+ Mock Tests)
Click Here to Avail Electronics Engineering Test Series (150+ Mock Tests)
Click Here to Avail Electrical Engineering Test Series (193+ Mock Tests)
If you aiming to crack GATE 2022, Other PSU Exams then you must try Online Classroom Program to get unlimited access to all the comprehensive courses and Mock Tests from the following links:
- ESE and GATE CE Online Classroom Program (25+ LIVE Courses and 150+ Mock Tests)
- ESE and GATE ME Online Classroom Program (24+ LIVE Courses and 160+ Mock Tests)
- ESE and GATE ECE Online Classroom Program (24+ LIVE Courses and 150+ Mock Tests)
- ESE and GATE EE Online Classroom Program (25+ LIVE Courses and 190+ Mock Tests)
- GATE CSE Online Classroom Program (21+ LIVE Courses and 100+ Mock Tests)
Download the BYJU’S Exam Prep App Now.
The most comprehensive exam prep app.
#DreamStriveSucceed
All the Best


