How to Solve LCM & HCF Questions? Tips & Tricks
By BYJU'S Exam Prep
Updated on: September 25th, 2023

Introduction of HCF
HCF stands for ‘Highest Common Factor’. To put simply, HCF of two (or more) numbers is the highest number that divides each of the numbers completely. For example in case of two numbers, 18 and 24, ‘6’ is their HCF. Why? Because no other number greater than ‘6’ divides 18 and 24 completely.
There is a rule that ‘any number that divides each of the two numbers, also divides their sum, their difference and the sum and difference of any multiples of two such numbers.
HCF can be calculated using following ways:
1) Prime Factorization: In this method, all the numbers are broken down to their prime factors. And, then common prime factors are multiplied to obtain HCF. To find the HCF of 1365, 1560 and 1755:
Note: Prime factors are the numbers which divide the number completely and are themselves prime numbers. Prime numbers are numbers that don’t come in the table of any number but 1. Like 11 is a prime number.
Let’s find prime factors of 1365, 1560 and 1755:
1365: 5×3×7×13
1560: 5×3×2×2×2×13
1755: 5×3×3×3×13
We can see the common Prime Factors which have equal number of frequency in each of the number are: 5, 3 and 13.
To get HCF, we’ll multiply these numbers. So, HCF = 5×3×13 = 195
So, 195 is the largest number that completely divides 1365, 1560 and 1755.
2) Division:
a) If we’ve to find HCF of two numbers: Divide greater number by smaller number. Then divide smaller number by remainder. Then, divide the remainder from last step by next remainder. Continue this cycle till remainder = 0. Last remainder to zero is our HCF.
To find HCF of 1365, 1560 and 1755.
First, we’ll find the HCF of 1365 and 1560 using the method described above.
Step I: 1560 ÷ 1365 ⇒ Remainder = 195
Step II: 1365 ÷ 195 ⇒ Remainder = 0
⇒ HCF of 1365 and 1560 = 195
Step III: 1755 ÷ 195 ⇒ Remainder = 0
So, HCF of 1365, 1560 and 1755 = 195
Other types of questions based on HCF:
(A) Find the greatest number which is such that when 76, 151 and 226 are divided by it, the remainders are all alike. Also, find the common remainder.
Let the common remainder be ‘k’
So, 76 – k, 151 – k and 226 – k are all divisible by that common number or HCF.
We know that if a number divides some numbers then it also divides their differences.
So, (151 – k) – (76 – k), (226 – k) – (151 – k) and (226 – k) – (76 – k) are also divisible by the HCF.
⇒ HCF divides 75, 75 and 150 also.
Therefore, the highest number that divides 75, 75 and 150 is their HCF
And, HCF of 75, 75 and 150 = 75
So, 75 divides 76 – k, 151 – k and 226 – k.
We can see on dividing 76 by 75 we get a remainder of 1 and 151÷75 gives remainder of 1.
So, k = 1
(B) The product of two numbers is 7168 and their HCF is 16; find the numbers.
Here, the two numbers must have HCF as their factors so two numbers can be 16a and 16b where ‘a’ and ‘b’ are prime factors of each number respectively.
Now, 16a×16b = 7168
⇒ ab = 28
If a=1 then b =28 ⇒ Numbers are 16 and 448
If a = 4 then b = 7 ⇒ Numbers are 64 and 112
If a = 2 then b = 14 ⇒ But 2 and 14 have ‘2’ in common among each other and we know that factors other than HCF don’t have anything in common among each other so it’s not possible.
So, the possible numbers are (16, 448) or (64, 112).
(C) In a school, 391 boys and 323 girls have been divided into the largest possible equal classes so that each class of boys numbers the same as each class of girls. What is the number of classes?
Number of classes = HCF of 391 and 323 = 17
Introduction of LCM
LCM stands for ‘Least Common Multiple’. To put simply, LCM of numbers is the least number which is completely divisible by each of the numbers. For example, 15 is divisible by 3 and 5.
Let’s see different methods for calculating LCM:
1) Prime factorization
Here, we multiply the prime factors with highest powers to get the LCM as shown below:
To get LCM of 18, 24, 60 and 150, we write down the prime factors with highest powers.
18 = 3×3×2 = 32×21
24 = 3×2×2×2 = 31×23
60 = 3×2×2×5 = 31×22×51
150 = 3×2×5×5 = 31×21×52
Here, the prime factors that occur in these numbers are 3, 2 and 5 and their highest powers are 32, 23 and 52
⇒ LCM = 32×23×52 = 1800
2) Basic Line division:
Here, we write numbers on RHS and on LHS we write a prime factor. We divide each of the number by the prime factor on LHS that divide different numbers. And, then we write the quotient, we get
when previous prime number completely divides numbers on RHS, in the next line. Now again we write a prime number and repeat the process until we get all the quotients as 1.

Here, the LCM is multiplication of all prime numbers on left
⇒ LCM = 23×32×52 = 1800
Other types of questions based on LCM
a) What least number must be subtracted from 1936 so that the remainder when divided by 9, 10, 15 will leave in each case the same remainder 7?
Step I: Find LCM of 9, 10 and 15 which is 90.
Step II: Divide 1936 by 90 ⇒ We get remainder as 46
Step III: From this remainder, we will subtract ‘7’ ⇒ 46 – 7 = 39
So, the required number is 39.
b) What is the least multiple of 7, which when divided by 2, 3, 4, 5 and 6 leaves the remainder 1, 2, 3, 4, 5 respectively?
Step I: Find LCM of 2, 3, 4, 5 and 6 which is 60
Step II: Number is 60k where k is a positive integer
Step III: Now, we’ll subtract respective remainders from numbers as 2 leaves remainder of 1 so 2 – 1 = 1; similarly, 3 – 2 = 1, 4 – 3 = 1, 5 – 4 = 1, 6 – 5 = 1
As remainder in each case is less than divisor by 1
⇒ Required number is (60k – 1)
Step IV: Since number is multiple of 7 so we’ll find the least value that will make the number divisible by 7.
When k = 1
60k – 1 = 60×1 – 1 = 59 ⇒ Not divisible by 7
When k = 2
60k – 1 = 60×2 – 1 = 119 ⇒ Divisible by 7.
c) The traffic lights at three different road crossing change after every 48 sec, 72 sec and 108 sec respectively. If they all change simultaneously at 8:20 hrs then at what time will they change again simultaneously?
LCM of 48, 72, 108 = 432
The traffic lights will change simultaneously after 432 seconds or 7m 12sec
∴ they will change simultaneously at 8:20:00 + 00:07:12 = 8:27:12 hrs.
Mixed questions based on HCF & LCM:
1) Product of two numbers = HCF×LCM (of both numbers)
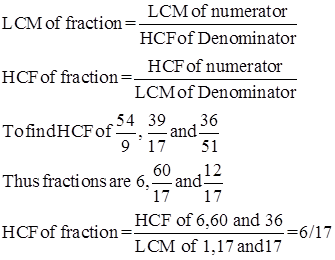
2) Fractions
3) Three men start travel around a circular path of 11 km. Their speeds are 4, 5.5 and 8 kmph respectively. When will they meet at the starting point?
Sol. Time taken by them to complete one revolution: (11/4), (11/5.5), (11/8) hrs respectively
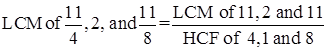
Thanks
Grade Stack Team


