Divide and Conquer Algorithm Study Notes
By BYJU'S Exam Prep
Updated on: September 25th, 2023

Divide and Conquer Design Technique
Divides the problem into smaller but similar subproblems (divide), solve it (conquer), and (combine) these solutions to create a solution to the original problem.
- Divide: Breaking the problem into several sub-problems that are similar to the original problem but smaller in size,
- Conquer: Solve the sub-problem recursively (successively and independently). If the subproblems are small enough, solve them in brute force fashion.
- Combine: Combine these solutions to sub-problems to create a solution to the original problem.
Table of content
Divide & Conquer Algorithms:
- Merge sort
- Quicksort
- Binary search
- Multiplication of large integers
- Matrix multiplication: Strassen’s algorithm
- Closest-pair algorithms
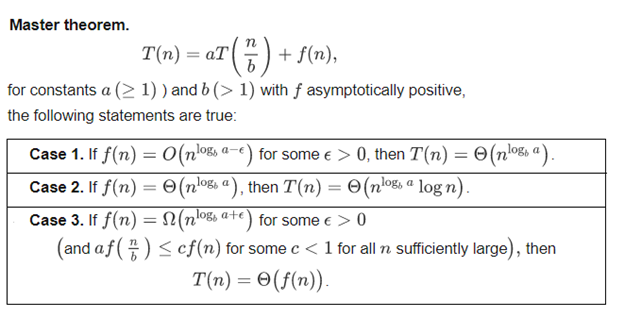
Divide & Conquer Algorithms can be solved using the master theorem to find the performance of algorithms.
Maximum and Minimum:
MaxMin(i, j, max, min)
{
if (i=j) then max := min := a[i];
else if (i=j-1) then
{
if (a[i] < a[j]) then max := a[j]; min := a[i];
else max := a[i]; min := a[j];
}
else
{
mid := ( i + j )/2;
// Solve the sub-problems.
MaxMin( i, mid, max, min );
MaxMin( mid+1, j, max1, min1 );
// Combine the solutions.
if (max < max1) then max := max1;
if (min > min1) then min := min1;
}
}
Time Complexity:
T(n) = 0; if n=1
= 1; if n=2
=2T(n/2)=2; if n>2
When n is a power of two, n = 2k, for some positive integer k, then
T(n) = 2T(n/2) + 2
= 2(2T(n/4) + 2) + 2
= 4T(n/4) + 4 + 2
…
= 2k-1 T(2) + ∑(1≤i≤k-1) 2k
= 2k-1 + 2k – 2
= 3n/2 – 2 = O(n)
Matrix Multiplication:
Simple approach of multiplication:
The product of two n × n matrices X and Y is a third n × n matrix Z = XY , with (i, j)th entry:
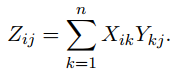
- Input: X, Y: Array(1 … n) of number
- Output: Z: Array(1 … n) := X x Y
- Obvious algorithm: Z has n2 entries, each of which multiples n pairs
- Zij=∑XikYkj
- Break X into X11, X12, X21, X22
- Break Y into Y11, Y12, Y21, Y22
- Break Z into Z11, Z12, Z21, Z22
- Let Z = product of two n/2 by n/2 arrays
Z11=X11×Y11+X12×Y21
Z12=X11×Y12+X12×Y22
Z21=X21×Y11+X22×Y21
Z22=X21×Y12+X22×Y22
- T(n)=Θ(1)+8T(n/2)+Θ(n2)
- Θ(1) time to partition matrices
- 8 Multiplications of arrays of size n/2n/2
- Θ(n2) time to add n×n matrices
- T(n)= Θ(n3) by master method
Strassen’s Algorithm:
Matrix multiplication is particularly easy to break into subproblems, because it can be performed block wise. Here divide X into four n/2 × n/2 blocks, and also Y:
![]()
Therefore, the product of X and Y is as follows:
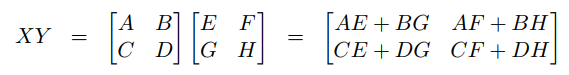
- Uses 7 rather than 8 multiplications of n/2 by n/2 arrays
- Has more additions and subtractions of n/2 by n/2 arrays
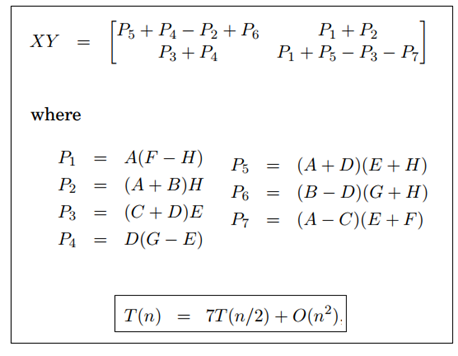
- T(n)=7T(n/2)+Θ(n2) if n>1
- Solution (by Master Method): T(n)=Θ(n log 7) =Θ(n2.81)
- Note: There are other matrix multiplication algorithms which can run even faster with T(n)=O(n2.38)
Maximal Subarray:
Divide and conquer approach to find the maximal subarray:
maxsub(int[] S; low, high: int)
Closest Pair Algorithm:
Input: P = {p(1), p(2) ,…, p(n)} where p(i) = ( x(i), y(i) ). A set of n points in the plane.
Output: The distance between the two points that are closest.
Note: The distance DELTA( i, j ) between p(i) and p(j) is defined by the expression:
Square root of { (x(i)-x(j))2 + (y(i)-y(j))2 }
float function closest_pair (P: point set; n: integer )
float DELTA-LEFT, DELTA-RIGHT;
float DELTA;
begin
if n = 2 then
return distance from p(1) to p(2);
else
P-LEFT := ( p(1), p(2) ,…, p(n/2) );
P-RIGHT := ( p(n/2+1), p(n/2+2) ,…, p(n) );
DELTA-LEFT := closestpair( P-LEFT, n/2 );
DELTA-RIGHT := closestpair( P-RIGHT, n/2 );
DELTA := minimum ( DELTA-LEFT, DELTA-RIGHT );
/* Determine whether there are points p(l) in P-LEFT and p(r) in P-RIGHT with
distance( p(l), p(r) ) < DELTA. If there are such points, set DELTA to be the smallest
distance.*/
return DELTA;
end if;
end closest_pair;
The section between the two comment lines is the `combine’ stage of the Divide-and-Conquer algorithm.
If there are points p(l) and p(r) whose distance apart is less than DELTA then it must be the case that
- The x-coordinates of p(l) and p(r) differ by at most DELTA.
- The y-coordinates of p(l) and p(r) differ by at most DELTA.
Integer Multiplication:
Multiplying two n-bit integers algorithm using divide and conquer approach is given below:

Summary:
|
Algorithm |
Worst-case running time |
Best-case running time |
Average-case running time |
|
Selection sort |
Θ (n2) |
Θ (n2) |
Θ (n2) |
|
Insertion sort |
Θ (n2) |
Θ (n) |
Θ (n2) |
|
Merge sort |
Θ (n log n) |
Θ (n log n) |
Θ (n log n) |
|
Quicksort |
Θ (n2) |
Θ (n log n) |
Θ (n log n) |
|
Strassen’s Matrix Multiplication |
Θ(n2.81) |
Θ(n2.81) |
Θ(n2.81) |
|
Matrix Multiplication |
Θ(n2.38) |
Θ(n2.38) |
Θ(n2.38) |
|
Integer Multiplication |
Θ(n1.585) |
Θ(n1.585) |
Θ(n1.585) |
|
Closest Pair |
Θ (n log n) |
Θ (n log n) |
Θ (n log n) |
|
Binary Search |
Θ(log n) |
Θ(log n) |
Θ(log n) |
|
Maximum and Minimum |
Θ(n) |
Θ(n) |
Θ(n) |
Candidates can also practice 110+ Mock tests for exams like GATE, NIELIT with BYJU’S Exam Prep Test Series check the following link:


