- Home/
- GATE ELECTRICAL/
- GATE EE/
- Article
Time Domain Analysis Study notes Part-1
By BYJU'S Exam Prep
Updated on: September 25th, 2023

Time domain analysis is a fundamental concept in signal processing and engineering, involving the examination of signals or systems in the time dimension. It primarily deals with the study of signals and their behavior over time. In time domain analysis, various properties and characteristics of a signal or a system are analyzed by observing its behavior in the time domain.
In this article, you will find the study notes on Time Domain analysis which will cover the topics such as Different Types of Input Signals, Time Response of Ist Order System, Time Response of Second Order System, Overdamped System Underdamped System, Undamped System & Critically Damped System. These notes are sure to help you prepare for engineering related exams like GATE, ISRO, ESE, and more.
Download GATE Electrical Engineering Revision Sheet and Formulae PDF
Time Domain Analysis
The Time Domain Analyzes of the system is to be done on basis of time. The analysis is only be applied when nature of input plus mathematical model of the control system is known. Expressing the main input signals is not an easy task and cannot be determined by simple equations. There are two components of any system’s time response, which are: Transient response & Steady state response.
- Transient Response: This response is dependent upon the system poles only and not on the type of input & it is sufficient to analyze the transient response using a step input.
- Steady-State Response: This response depends on system dynamics and the input quantity. It is then examined using different test signals by final value theorem.
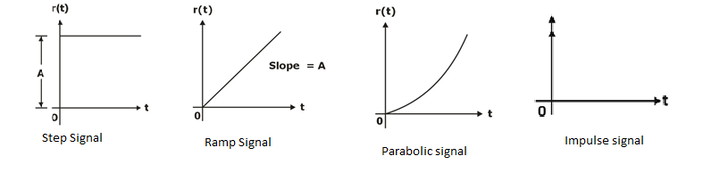
Standard Test Input Signals
- Step signal: r(t) = Au(t).
- Ramp signal: r(t) = At; t > 0.
- Parabolic signal: r (t) At2/2; t > 0.
- Impulse signal: r(t) = δ(t).
Initial value of the response [c(0)]
Initial value theorem is applied only when the number of poles of C(s) is more than the number of zeros i.e. function C(s) must be strictly proper.
Final value of the Response [c(∞)] :-
Final value theorem: – Final value theorem is applied when all the poles of S C(s) lie in the left half of the S-plane
Time-Response of First-Order System
A first order control system is one for which the highest power of ‘s’ in the denominator of its transfer function is equal to 1.Thus, a first order control system is expressed by a transfer function 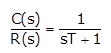
Also, the block diagram representation of the above expression is shown in the below.
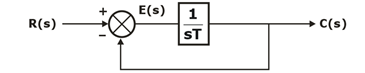
Download Formulas for GATE Electrical Engineering – Signals and Systems PDF
Unit step input of a first order system:
The step response of a dynamic system measures how the dynamic system responds to a step input signal.
The output for the system is expressed as, C(s) = R(s)
As the input is a unit step function, r(t) = 1 and R(s) = 1/s
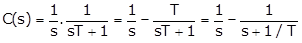
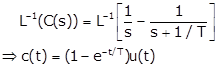
The error is given by, e(t) = r(t) – c(t) = e–t/T. u(t)
The steady state error,
![]()
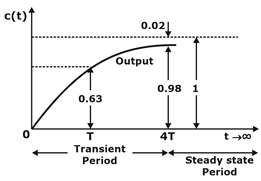
The time response in relation to above equation is shown in the figure.
Ramp response of first-order system:
As the input is a unit ramp function, r(t) = t and R(s) = 1/s2
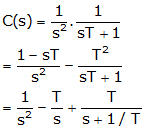
Taking inverse Laplace transform on both sides,

C(t) = (t – T + Te–t/T) u(t)
The error is given by e(t) = r(t) – c(t) = (T – Te–t/T) u(t)
The steady state error is
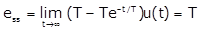
The time response in relation to the above equation is shown in the figure.
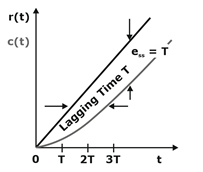
The time response shown in figure indicates that during steady state, the output velocity matches with the input velocity but lags behind the input by time T and a positional error of T units exists in the system.
Thus, the first-order system will track the unit ramp input with a steady-state error T, which is equal to the time-constant of the system.
Unit impulse response of a first order control system:
The impulse response of a dynamic system measures how the system responds to an impulse input signal.
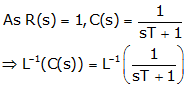
⇒ c(t) = (1/T)e–t/T
The error is given by e(t) = r(t) – c(t)
The steady state error is
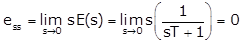
TIME RESPONSE OF SECOND ORDER CONTROL SYSTEM
Second order systems are important for several reasons.
- They are the simplest systems that exhibit oscillations and overshoot.
- Many important systems exhibit second order system behavior.
- Second order behavior is part of the behavior of higher order systems and understanding second order systems helps you to understand higher order systems.
A second order control system is one for which the highest power ‘s’ in the denominator of its transfer function is equal to 2.
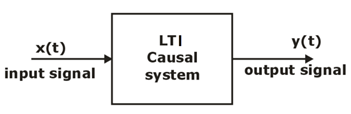
Consider a linear time-invariant system where input to the system is given by x(t) & its corresponding output as y(t).
The general differential equation of second order system is given as
 is the transfer function of 2nd order system.
is the transfer function of 2nd order system.
The characteristic equation of the second order system is
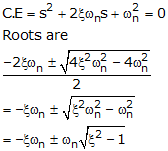
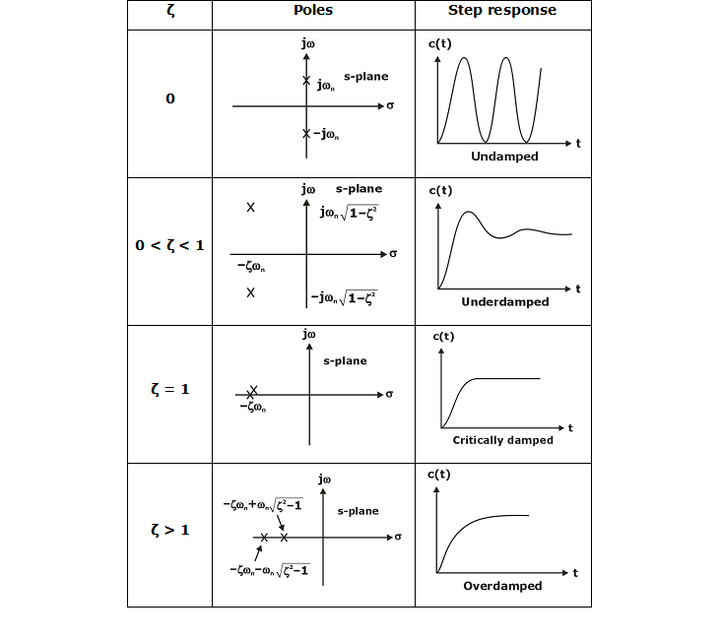
Case 1: ξ = 0
In this case, the poles of the system lie on jω axis and hence the system is said to be undamped system.
Case 2: ξ = 1
In this case, the roots or poles of the system is always real and equal and equals to –ωn i.e. s1, s2 = – ωn
Therefore, the system is said to be critically damped system.
Case 3: 0 < ξ < 1
In this case, the poles of the system are complex conjugate to each other. i.e.

Hence, the system is called under damped system.
Case 4: ξ > 1
In this case, the roots or poles of the system are real but unequal i.e.,
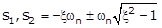
Therefore, the system is said to be over damped system.
Output Response & Pole-Zero Plot of Various Forms of Systems:
Download Formulas for GATE Electrical Engineering – Electrical Machines PDF
If you are preparing for GATE and ESE, avail Online Classroom Program to get unlimited access to all the live structured courses and mock tests from the following link:



