Tips to Prepare Trigonometry for NDA exam

With the help of this post, we will help the aspirants understand how they should prepare trigonometry for the mathematics section of the NDA exam. So, read these NDA trigonometry preparation tips now to score full marks in this topic. We have also covered the important trigonometric identities for the NDA exam, which the students must remember.

The subtopics in trigonometry are angles and their measure in degree and in radians, trigonometric ratios, sum and difference formulae, trigonometric identities, multiple and sub-multiple angles, properties of a triangle, inverse trigonometric functions, and applications of trigonometry.

The candidate should be well informed about the correct syllabus, that is, the topics and sub-topics and the marks distribution or weightage, before proceeding with his study plan to avoid any confusion. Solving at least 70% of the mathematics section with 90% accuracy will be beneficial for the candidate.

How to prepare Trigonometry for the Mathematics section of NDA exam

One can follow the under-mentioned tips for proper preparation of the trigonometry in the maths section of the Combined Defense Services exam!

1. Memorize certain trigonometric values

The candidate should try remembering trigonometric values such as Sin 18, Cos 18, Sin 36, Cos 36, etc. In addition to this, he should also memorize the trigonometric values of angles such as 0, 30, 45, 60, 90 degrees.

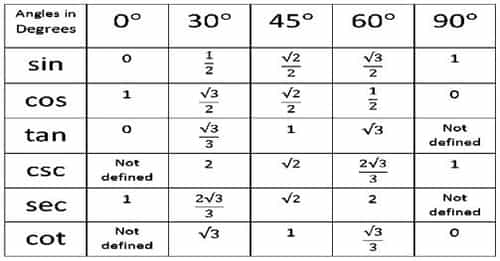
2. Opt for shortcuts

• Trigonometry is one of those topics wherein shortcuts are extremely handy.
• The candidate must learn various tricks and shortcuts for better time management.
• For instance, to remember the table of trigonometric values, the candidate must only memorize the values of Sin 0, Sin 30, Sin 45, Sin 60, Sin 90. All other values for those angles, that is, Cos, Tan, Cot, Sec, and Cosec can be derived from the sine value as given below:

• Tan A = Sin A / Cos A
• Cot A = 1 / Tan A
• Sec A = 1 / Cos A
• Cosec A = 1 / Sin A

3. Prepare a weekly schedule ahead of every week

• Mathematics is a subject that one can get a good command over by practising it daily.
• The candidate should prepare the entire week’s schedule in advance.
• He should ensure that at least one sub-topic each from all the high weightage topics, including trigonometry, is solved daily in order to ensure consistency and to avoid losing touch.
• The candidate should start with the difficult topics first if he has more time; else he should start by strengthening those topics that he finds easier and then move on to the difficult ones and look for shortcuts to understand them.

4. Prepare a revision sheet for formulae

• The candidate should prepare a revision sheet by writing down all the trigonometric formulae in a systematic manner to aide in quick referencing and last-minute preparations.

5. Solve mock papers and previous year's question papers

• Solving mock papers will give the candidates an estimate of the amount of time to be spent per question and also help the candidate decide if he needs to work on his time management.
• Previous years’ question papers will help the candidate get an idea about the paper pattern and the weightage given to each sub-topic under trigonometry.
• A regular NDA & NA paper has about 10-15 questions from trigonometry, with a majority of weightage being usually given to measurement of angles and trigonometric ratios.
• The candidate should be well informed about the context and sub-topic that these questions are derived from.
• Test simulators are another way to get a real-time experience of the NDA and NA examination. These simulations ensure that the candidates get a feel of the exam beforehand.

6. Approximations and calculations

• The candidate should work on improving his calculations by practising mental arithmetic and quick math.
• This includes memorizing squares and cubes of the first 10-15 digits, multiplication tables to 25, etc.
• The candidate should also use approximations wherever possible. If the values given in the options deviate to a great degree, approximation comes in handy to get the solution faster.
• However, if the values given in the options do not deviate much, the candidate should use the accurate values for calculation.

7. Use reference books or guides

While NCERT books give a brief but comprehensive idea about each topic, other reference books can also be used for a more immersive and in-depth information about various topics. We have already shared the list of useful books for different sections of the NDA exam. Go through it to know the sources you can refer to prepare this topic.

Important Trigonometric Identities for NDA Exam

• Sin² A + Cos² A = 1
• 1 + Tan² A = Sec² A
• 1 + Cot² A = Cosec² A
• Sin (A+B) = Sin A Cos B + Cos A Sin B
• Sin (A-B) = Sin A Cos B – Cos A Sin B
• Cos (A+B) = Cos A Cos B – Sin A Sin B
• Cos (A-B) = Cos A Cos B + Sin A Sin B
• Tan (A+B) = (Tan A + Tan B) / (1 – Tan A Tan B)
• Tan (A-B) = (Tan A – Tan B) / (1 + Tan A Tan B)
• Cot (A+B) = (Cot A – Cot B) / (1 – Cot A Cot B)
• Cot (A-B) = (Cot A + Cot B) / (1 + Cot A Cot B)
• Sin 2A = 2 Sin A Cos A
• Cos 2A = Cos² A – Sin² A = (2 Cos² A – 1) = (1 - Sin² A)
• Tan 2A = 2 Tan A/ (1 – Tan² A)
• Sin A Cos B = ½ (Sin (A+B) + Sin (A-B))
• Cos A Sin B = ½ (Sin (A+B) - Sin (A-B))
• Cos A Cos B = ½ (Cos (A+B) + Cos (A-B))
• Sin A Sin B = ½ (Cos (A-B) - Cos (A+B))
• Sin 3A = 3 Sin A – 4 Sin³ A
• Cos 3A = 4 Cos³ A – 3 Cos A
• Tan 3A = (3 Tan A – Tan³ A) / (1 – 3 Tan² A)

All the best for your exams,