Heat-Transfer : Free and Forced Convection

Free and Forced Convection:

Convection: Convection is the mechanism of heat transfer that occurs between a solid surface & the surrounding fluid in the presence of bulk fluid motion.

Convection is classified as Natural (or free) and Forced convection depending on how the fluid motion is initiated.

The rate of convection heat transfer is expressed by Newton’s law of cooling and it is given as  Forced Convection

Where h is the heat transfer coefficient and A is the area of the contact.

The convective heat transfer coefficient h strongly depends on the fluid properties and roughness of the solid surface, and the type of the fluid flow (laminar or turbulent).

h = f(ρ, V, D, μ, C_p, K)

Boundary layer:

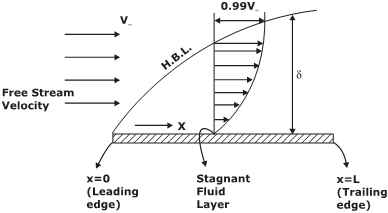
Hydrodynamic Boundary Layer:

Hydrodynamic Boundary Layer is defined as a thin region formed on the plate inside which velocity gradients are seen in a normal direction to the plate.

(at any x measured from leading edge)

If Re_x < 5 × 10⁵, then flow is LAMINAR

If Re > 6.5 to 7 × 10⁵, Then flow is TURBULENT

CASE 1: Flow over flat plates:

Hydrodynamic boundary layer:

δ → Thickness of Hydrodynamic Boundary Layer at any distance x measured from leading-edge [i.e., x = 0] of the plate.

δ = f(x)

Thermal boundary layer:

Similar to velocity boundary layer, a thermal boundary layer develops when a fluid at specific temperature flows over a surface which is at different temperature. 

 T = f (x,y)

δ_t = Thickness of thermal Boundary Layer

δ_t = f(x)

The Thermal boundary layer thickness is defined as the distance measured form the solid boundary in y direction at which 

Thermal boundary layer (T_∞ > T_w):

Energy balance for thermal boundary layer in a control volume:

T = f (x, y)

Assuming steady-state conditions for the control volume,

dq = Heat conducted through the stagnant fluid layer at y = 0 = Heat convected between the plate & free stream fluid

Where, h_x = Local convective heat transfer coefficient at that x,

variation of h_x with x :

Nusselt Number (Nu):

Prandtl Number (Pr):

Peclet Number (Pe):

It is the product of Reynold number and Prandtl number.

Peclet number = Reynold number × Prandtl number

Stanton Number (St): 
 It is the ratio of nusselt number to the Peclet number.

Relation between thermal boundary layer and hydrodynamic boundary layer:

Reynold Colburn analogy:

Flow Over Flat Plate:

Laminar Flow: 

Local Nusselt number at the location x for laminar flow over a flat plate is,

Average Nusselt number for the entire length of the plate,

The local friction coefficient at the location x for laminar flow over a flat plate is

where x is the distance from the leading edge of the plate and 

 Average local friction coefficient for entire length of the plate

Critical Reynolds number for flow over flat plate is 5×10⁵

Turbulent Flow:  

Local Nusselt number at location x for turbulent flow over a flat isothermal plate are: 

 Average Nusselt number:

Local friction coefficient:

Average friction coefficient:

Pipe Flow:

Laminar Flow: 

The bulk mean temperature (T_m) at a given cross-section of the pipe of flowing fluid is defined as the constant temperature which takes into account the variation of temperature of fluid layers with respect to radius at that cross-section of the pipe and hence indicates the total thermal energy or enthalpy carried by the fluid through that cross-section.

Constant heat flux:

No dependence on Re and Pr.

Constant surface Temperature:

Turbulent Flow:  

n = 0.3 for cooling and n = 0.4 for heating

Free Convection or Natural Convection:

No velocity evident but the flow occurs due to buoyancy forces arising out of density changes of fluid.

Free convection over different geometries:

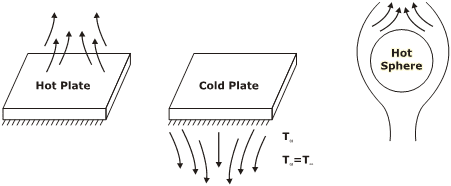
In any free convection heat transfer,

h = f (g, β, ΔT, L, μ, ρ, C_p, k)

μ, ρ, Cp, k = Thermophysical Properties of fluid

β = Isobaric volume expansion coefficient of fluid [per kelvin]

Characteristic dimension for various dimensionless numbers:

For vertical plates & vertical cylinders.

For horizontal plate      for horizontal cylinder

Grashof Number (Gr): 

where 

g = gravitational acceleration, m/s² 

β = coefficient of volume expansion, 1/K 

δ = characteristic length of the geometry, m 

ν = kinematics viscosity of the fluid, m²/s  

Grashof number (Gr) replaces Reynolds Number (Re) in free convection heat transfer.

Therefore, in any free convection heat transfer,

Heat transfer coefficient:

Nu = f (Gr Pr)

product of Gr & Pr is called Rayleigh Number (Ra)

Nu = C (Gr Pr)^m

C & m are constants which vary from case to case.

m = 1/4 for Laminar Flow

m = 1/3 for Turbulent Flow

The flow during free convection heat transfer is decided as Laminar or Turbulent based on the value of (Gr Pr) product.

If Gr Pr < 10⁹ Then, flow is Laminar

If Gr Pr > 10⁹ Then, flow is Turbulent

You can avail of BYJU’S Exam Prep Online classroom program for all AE & JE Exams:

BYJU’S Exam Prep Online Classroom Program for AE & JE Exams (12+ Structured LIVE Courses)

You can avail of BYJU’S Exam Prep Test series specially designed for all AE & JE Exams:

BYJU’S Exam Prep Test Series AE & JE Get Unlimited Access to all (160+ Mock Tests)

Thanks

Team BYJU’S Exam Prep

Download  BYJU’S Exam Prep APP, for the best Exam Preparation, Free Mock tests, Live Classes.