Fluid mechanics : Boundary-Layer & Forces on Submerged Body

Introduction

Boundary layer phenomenon occurs when a fluid flows over a flat plate causing laminar or turbulent flow. This topic defines various parameters such as Energy thickness, Momentum thickness, Boundary layer thickness etc.

Boundary layer theory

• Boundary layer theory was developed by Prandtl in the year 1904.
• When a real fluid flows over a solid body, the velocity of fluid at the boundary will be zero. If boundary is stationary.
• As we move away from boundary in perpendicular direction, velocity increases to the free stream velocity. It means velocity gradient  will exist.

Note: velocity gradient  does not exist outside the boundary layer as outside the boundary layer velocity is constant and equal to free stream velocity.

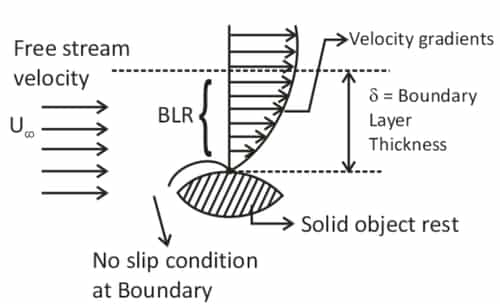
Boundary Layer Thickness (δ): It is the distance from the boundary to the point where velocity of fluid is approximately equal to 99% of free stream velocity. It is represented by δ.

Development of Boundary Layer: Development of boundary layer can be divided in three regions: laminar, transition, turbulent.

Boundary conditions:

(i) at y = 0, u = 0

(ii) y = δ, u = u_∞

(iii) y = δ, =0

(iv) x = 0, δ = 0

Here, x is distance from leading edge in horizontal direction.

Reynolds number (R_e) 

For laminar boundary layer (Re)_x < 5 × 10⁵ (For flat plate)

For turbulent boundary layer (Re)_x > 5 × 10⁵ (For flat plate)

Displacement Thickness (δ*): It is observed that inside the boundary layer velocity of fluid is less than free stream velocity hence, discharge is less in this region. To compensate for reduction in discharge the boundary is displaced outward in perpendicular direction by some distance. This distance is called displacement thickness (δ*).

Momentum Thickness (δ**  or θ): As due to boundary layer reduction in velocity occurs, so momentum also decreases. Momentum thickness is defined as the distance measured normal to boundary of solid body by which the boundary should be displaced to compensate for the reduction in momentum of flowing fluid.

Energy Thickness (δe): It is defined as distance measured perpendicular to the boundary of solid body by which the boundary should be displaced to compensate for reduction in kinetic energy of flowing fluid (KE decreases due to formation of boundary layer)

Shape Factor(H):

• It is defined as the ratio of displacement thickness to momentum thickness.
• Hence: H=δ*/θ
• This ratio depends solely on the shape of the velocity profile.

For flow inside pipe flow will be turbulent, If Re > 4000

for flat plate flow will be turbulent, If Re > 5 × 105

Von Karman Momentum Integral Equation

where, θ = momentum thickness; ρ = Density of fluid.

This is applied to:
1. Laminar boundary layers,
2. Transition boundary layers, and
3. Turbulent boundary layer flows.

Shear stress: 

where, U = Free stream velocity

Local Coefficient of Drag (C_D^*): It is defined as the ratio of the shear stress τ₀ to the quantity 

It is denoted by 

Average Coefficient of Drag (C_D): It is defined as the ratio of the total drag force to 

It is denoted by

Where, A = Area of surface, U = Free stream velocity, ρ = Mass density of fluid.

Total drag on a flat plate due to laminar and turbulent boundary layer:-

Total drag= Laminar drag upto transition boundary + turbulent drag for whole plate - turbulent drag upto transition boundary

Drag force=F_D = ½ ρ × v2 × C_D ×A

Blassius Experiment Results

where x = Distance from leading edge

Re_x = Reynold number for length x.

Re_L = Reynold number at end of plane

For laminar flow, Boundary layer thickness  δ ∝ √x

                         Shear stress at solid surface 

Velocity profile for turbulent boundary layer on Flate surface is

where n =1/7  for R_e < 10⁷ but more than 5 × 10⁵                            

Boundary Layer Separation:

Let us take curve surface ABCSD where fluid flow separation point S is determined from the condition 

If  the flow is separated 

If  the flow is on the verge of separation 

If  the flow will not separate or flow will remain attained 

Methods of Preventing Separation of Boundary Layer:

• Supplying additional energy from a blower.
• Providing a bypass in the slotted
• Rotating boundary in the direction of flow.
• Providing small divergence in diffuser.
• Providing guide blades in a bend.
• Providing a trip wire ring in the laminar region for the flow over a sphere.

You can avail of BYJU’S Exam Prep Online classroom program for all AE & JE Exams:

BYJU’S Exam Prep Online Classroom Program for AE & JE Exams (12+ Structured LIVE Courses)

You can avail of BYJU’S Exam Prep Test series specially designed for all AE & JE Exams:

BYJU’S Exam Prep Test Series AE & JE Get Unlimited Access to all (160+ Mock Tests)

Thanks

Team BYJU’S Exam Prep

Download  BYJU’S Exam Prep APP, for the best Exam Preparation, Free Mock tests, Live Classes.