An important part of the Defence exam is Mathematics, it is asked in CDS (IMA, INA & AFA), AFCAT, NDA & other exams like Air Force Group X & Y. Some students find Maths very difficult, so to help them here we are discussing an important maths topic - Trigonometry (Heights & Distances).
This part, we will try to solve questions with the help of ratio. This trick will save a lot of time and useful during the exam period. This article also contains some useful formula.
Defence Exam Notes: Heights & Distance
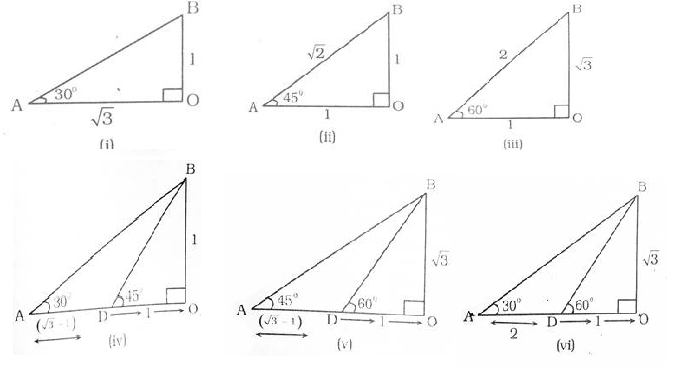
Here are some ratio figure which you have to remember
Important short tricks are :
Note: ![]() only when the sum of angle i.e
only when the sum of angle i.e ![]()

Some Important questions are as follows:
Example 1: The angle of elevation of the top of a tower at a distance of 500 m from its foot is 30°. The height of the tower is :
(c)500
Ans. (d)
Short trick:
Solve it with ratio, as the angle of elevation is 30° then ratio between P: B: H is 1:√3:2 so √3= 500 then 1= 500/√3 and height is equal to ![]()
Example 2: The banks of a river are parallel. A swimmer starts from a point on one of the banks and swims in a straight line inclined to the bank at 450 and reaches the opposite bank at a point 20 m from the point opposite to the starting point. The breadth of the river is :
(a) 20 m
(b) 28.28 m
(c) 14.14 m
(d) 40 m
Ans. (c) 14.14 m
Solution:
Let A be the starting point and B, the endpoint of the swimmer. Then AB = 20m & ![]()
Short Method;
AS the angle of elevation is 45° then the ratio of P: B: H i.e. 1:1:√2
here √2 =20 then 1 =20/√2
Question 3: A man from the top a 50m high tower, sees a car moving towards the tower at an angle of depression of 300. After some time, the angle of depression becomes 600. The distance (in m) travelled by car during this time is –
Ans. (c)
Solution:
AB = AC – BC
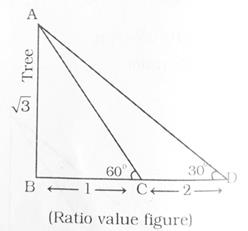
Example 4:A person standing on the bank of a river observes that the angle of elevation of the top of a tree on the opposite side of the bank is 600. When he moves 50m away from the bank, the angle of elevation becomes 300. The height of the tree and width of river respectively are :
(c) ![]()
(d) None of these
Answer: c)
Solution:
Ratio value original value
height of the tree= h (ratio value = ![]() )=
)=![]()
and width of the river = x (ratio value = 1) = 25 m
Example 5: From the top of a pillar of height 80 m the angle of elevation and depression of the top and bottom of another pillar are 300 and 450 respectively. The height of second pillar (in metre) is:
Answer: (c)
Solution:
Let AB and CD are pillars.
Let DE = h
Required height
Example 6: Two poles of equal height are standing opposite to each other on either side of a road, which is 28m wide. From a point between them on the road, the angles of elevation of the tops are 300 and 600. The height of each pole is:
Ans. (d)
Let AB and CD be the pole and AC be the road.
Let AE = x, then EC = 28-x and AB = CD = h. Then let AB = CD=√3
then, EC =1 and AE = 3
AC (ratio value) = 3 + 1 = 4
4 = 28 then 1 =7
and √3=7√3 so height of tower is 7√3.
Example 7: There are two vertical posts, one on each side of a road, just opposite to each other. One post is 108 metre high. From the top of this post, the angles of depression of the top and foot of the other post are 300 and 600 respectively. The height of the other post is :
(a)36
(b)72
(c)76
(d)80
Ans (b)
The height of greater Lower i.e. AB = 108 = H
so height of tower is 72
Example 8: An aeroplane when flying at height of 5000 m from the ground passes vertically above another aeroplane at an instant, when the angles of elevation of the two aeroplanes from the same point on the ground are 600 and 450 respectively. The vertical distance between the aeroplanes at that instant is:
(d)4500 m
Ans (c)
In this question we have two triangle ABC and triangle DBC. In triangle ABC we apply the ratio according to 60° and in triangle DBC we apply ratio according to the 45°. That why we take AB=√3 and DB =1.
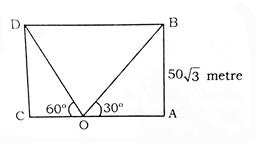
Example 9: A boy standing in the middle of a square field which is of length 50√3 m, observes a flying bird in the north at an angle of elevation of 300 and after 2 minutes, he observes the same bird in the south at an angle of elevation of 600. If the bird flies all along in a straight line at a height of then its speed in km/h is:
(a) 4.5
(b) 3
(c) 9
(d) 6
Ans.(d)
In ABO
According to the ratio method
From triangle DCO
DO cot AO = 150 + 50 = 200 m
Example 10: A tree is broken by the wind. If the top of the tree struck the round at an angle of 300 and at a distance of 30 m from the root, then the height of the tree is :
Ans. (b)
√3=30
1= 10√3 & 2 =20√3
so total height is 1+2 =10√3+20√3= 30√3
Example 11: The angle of elevation of a cloud from height h above the level of water in a lake is a and the angle of the depression of its image in the lake is b. Then, the height of the cloud above the surface of the lake is :
Ans. (d)
Let P be the cloud at height H above the level of the water in the lake Q its image in the water
B is at a point at a height AB = h, above the water, Angle of elevation of P and depression of Q from B are respectively
In triangle PBM
From equations (i) and (ii),
More from us:
Important Study Notes for Defence Exams
BYJU'S Exam Prep Hall of Fame
Thanks
Download the BYJU’S Exam Prep App Now
The Most Comprehensive Exam Prep App
#DreamStriveSucceed






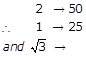







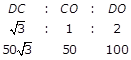








Comments
write a comment