Theory of Errors
By BYJU'S Exam Prep
Updated on: September 25th, 2023

The theory of errors is a very important topic of Civil Engineering that comes under surveying subjects. In this subject, different types of survey works are carried out, hence the chance of errors being made. So it is important to understand the different theories of errors. Knowing these theories, we can apply them and correct the observed value.
Theory of Errors PDF
In the theory of errors topic, different theories can be used to correct the observed value taken by an instrument. With the help of these theories, an observed reading can be corrected, which are given by many mathematical interventions. This article contains basic notes on the “Theory of Errors” topic of the “Surveying” subject.
Table of content
What is the Meaning of Theory of Errors?
The theory of errors explains different theories that can be used to correct the incorrect observed readings. The theory of errors can be applied weight of observed readings. With the help of different theories, mean errors, most probable errors, etc., can be applied. In surveying, errors can be classified as systematic errors, instrument errors, etc.
In surveying works, length, area, etc., need to be measured, and it may consist of some errors in the measurement because no instrument can be perfect. So, understanding the theory of errors is very important to rectify those errors in the measurement. These errors may be due to instrumental errors or due to improper measurements.
Surveying Errors and Their Adjustments
Errors can occur due to improper measurements taken by the surveyor or instrumental errors. These errors must be adjusted in the observed readings for the correct measurement. This adjustment is only possible by knowing the different types of errors and their source of generation.
Source of Errors
As per the GATE CE syllabus, errors in surveying may arise from three main sources:
- Instrumental: Surveying errors may arise due to imperfection or faulty instrument adjustment with which measurement is being taken. For example, a tape may be too long, or an angle measuring instrument may be out of adjustment. Such errors are known as instrumental errors.
- Personal: Error may also arise due to wanting perfection of human sight in observing and touch in manipulating instruments. For example, an error may occur in taking the level reading or reading and angle on the circle of a theodolite. Such errors are known as personal errors.
- Natural: Error in surveying may also be due to variations in natural phenomena such as temperature, humidity, gravity, wind, refraction and magnetic declination. If they are not properly observed while taking measurements, the results will be incorrect. For example, a tape may be 20 meters at 20°C, but its length will change if the field temperature is different.
Types of Errors in Surveying
As per the theory of errors, in a survey, work errors can be caused due to various reasons. It may be due to errors in instruments or due to errors in measurements. Ordinary errors in surveying met within all classes of survey work may be classified as:
- Mistakes
- Accidental errors
- Systematic or cumulative errors
- Compensating errors
Mistakes: Mistakes arise from inattention, inexperience, carelessness and poor judgment or confusion in the observer’s mind. They do not follow any mathematical rule (law of probability) and may be large or small, positive or negative. In the theory of errors, mistakes are also known as gross errors, and they cannot be measured.
Accidental Errors: Surveying errors can occur due to unavoidable circumstances like variations in atmospheric conditions, which are entirely beyond the control of the observer. Errors in surveying due to imperfection in measuring instruments and even imperfection of eyesight fall in this category. They may be positive and may change signs. They cannot be accounted for.
Systematic or Cumulative Errors: A systematic or cumulative error is an error that, under the same conditions, will always be of the same size and sign. A systematic error always follows some definite mathematical or physical law, and correction can be determined and applied. Their effect is, therefore, cumulative. As per the theory of errors, comparing random vs systematic errors will help candidates understand these concepts better for the GATE exam. For example, if a tape is P cm short and is stretched N times, the total error in the length measurement will be P.N cm.
Systematic errors may arise due to (i) variations of temperature, humidity, pressure, current velocity, curvature, refraction, etc. and (ii) faulty setting or improper levelling of any instrument and personal vision of an individual. The following are the examples:
- Faulty alignment of a line
- An instrument is not levelled properly
- An instrument is not adjusted properly
Compensating Errors: This type of surveying error tends to occur in both directions, i.e., the errors may sometimes be positive and sometimes negative, thereby compensating each other. They sometimes tend in one direction and sometimes in the other, i.e., they are equally likely to make the apparent result large or small. The following are a few examples:
- The discrepancy between chain and tape measurements when both are used simultaneously.
- Inaccuracy in marking chain lengths on the ground.
- Inaccurate centring.
- Inaccurate bisection of an object.
Propagation of Errors
Measurements are used for the calculation of different parameters. As per the theory of errors, the measurements are fraught with errors, it is important to know how these errors combine in various mathematical operations. This propagation of errors is also considered important for the GATE exam, as NAT questions may be asked from this topic.
Error Propagation in a Sum or Difference of Measurements
When two or more quantities are added or subtracted, the error in result (Es) is the square root of the sum of the square of the errors (e1, e2, …..) of the individual quantity, i.e.,

Error Propagation in a Product of Measurements
As per the theory of errors, when two or more quantities are multiplied, the error in the result (Eproduct) is the square root of the sum of the square of the fractional errors of the individual quantity. Thus
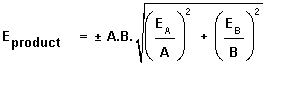
, EA and EB are errors in observed values of A and B, respectively.
For example, A rectangle is measured 160.881 ± 0.026 cm long and 75.007 ± 0.001 cm wide. The error in its area (12,067 cm2) is
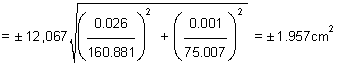
Error Propagation in a Division of Measurements
As per the theory of errors, when two or more quantities are divided, the error in the result is the square root of the sum of the square of the fractional errors in the individual quantity.
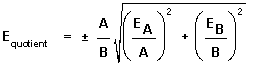
For example: If the area of a rectangular plot is somehow known to be 49,650 ± 10 m2 and the width dimension measured several times is found to be 175.66 ± 0.46 m, the calculated length dimension is
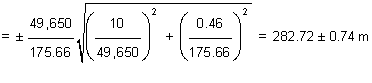
If you are preparing for ESE/ GATE or other PSU Exams (Civil Engineering), then avail Online Classroom Program for ESE and GATE CE.
Online Classroom Program for ESE/GATE CE
You can avail of BYJU’S Exam Prep Test Series, specially designed for all Civil Engineering Exams.



