- Home/
- GATE MECHANICAL/
- GATE ME/
- Article
Euler’s Theory of Columns: Limitations of Euler’s Theory
By BYJU'S Exam Prep
Updated on: September 25th, 2023

Euler’s Theory of Columns states that a column behaves to resist buckling. He stated that the end condition of the columns and flexural rigidity influence buckling. Therefore, Euler’s Theory of Columns can be used to calculate the buckling load of the column (critical after which the column buckles). There is also a condition that the column is elastic and stress remains constant.
Euler’s Theory of Columns PDF
Euler’s Theory of Columns plays a significant role while preparing for GATE ME 2023 Exam. Below we have given comprehensive Euler’s Theory of Columns Study Notes for Mechanical Engineering. Let’s take a look and understand the Theory deeply.
Table of content
Euler’s Theory of Columns
Euler’s theory of columns relates the buckling strength of a column to different types of column supports. According to Euler’s theory of column, failure of a column occurs either due to the buckling load or due to the buckling criteria.
Euler’s theory of columns is a very important concept for the designing of the column type member and also for the designing of the struts. This theorem is used to calculate the critical buckling load on the column.
Columns and Struts
Columns and struts are structural members used to resist compressive loads. Struts are only designed to resist the compressive load, but the column is designed to take a minimum of the compressive strength and buckling load.
- A structural member subjected to an axial compressive force is called a strut. Per definition, struts may be horizontal, inclined, or even vertical.
- The vertical strut is called a column.
Students can also Check this BYJUS Exam Prep Video Regarding Euler’s theory of Columns and Buckingham Theorem to better understand concepts.
Assumptions of Euler’s Column Theory
The assumptions of Euler’s theory are mentioned below.
- Axis of the column is perfectly straight when unloaded.
- The thrust line coincides exactly with the unstrained axis of the column.
- Flexural rigidity El is uniform.
- Material is isotropic and homogeneous.
Limitation of Euler’s Formula
The limitation of Euler’s theory of columns is the limiting conditions of a theory for that particular condition on which the theory fails. Here are a few points for which Euler’s theory becomes violated, and this condition is called the limitation of the theory.
- The column always has crookedness, and the load may not be exactly axial.
- This formula buckling takes into account the axial stress, and this formula gives the buckling load may be much more than the actual buckling(or crippling load)
- The maximum load at which the column tends to have lateral displacement or tends to buckle is known as buckling or crippling load. Load columns can be analyzed with the Euler’s column formulas can be given as
PE = π2EI/Le2
Where E = Modulus of elasticity, Le= Effective Length of the column, and I = Moment of inertia of column section.
For Both End Hinged
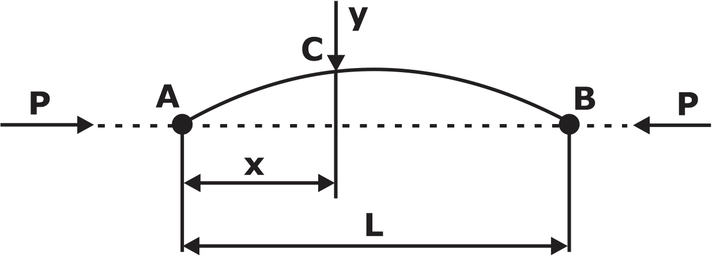
In the case of Column hinged at both ends, Le= L
PE = π2EI/L2
where
PE = allowable load
E = Modulus of elastisity(Pa (N/m2))
L = length of column (in, m)
I =Moment of inertia(in4, m4)
For One End Fixed and the Other Free
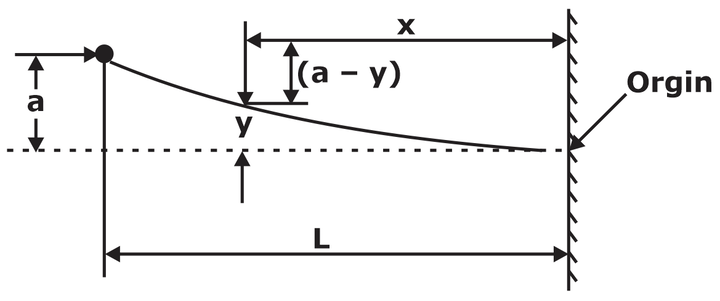
In the case of column, one end is fixed and the other free: Le= 2L
PE = π2EI/4L2
For Both Ends fixed
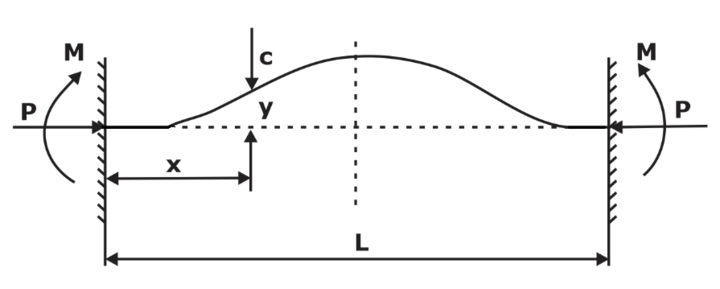
In the case of a Column with both ends, Fixed Le= L/2
PE = 4π2EI/4L2
For One End Fixed and the Other Hinged
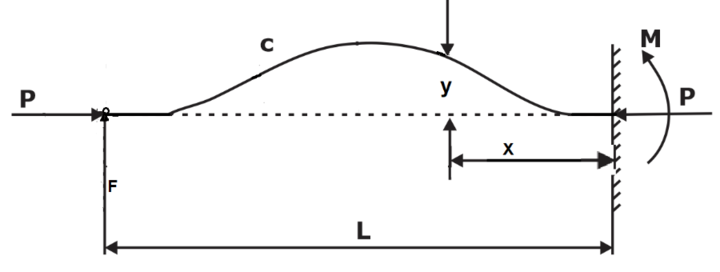
In the case of a Column with one end fixed and the other hinged, Le= L/√2
PE = 2π2EI/4L2
Effective Length for Different End Conditions
Effective length is the length of the column that is effectively used to resist compressive loads. The effective length depends on the type of end conditions. Here the value of the effective length for some end conditions is given below in the tabular form.
Slenderness Ratio (S)
The slenderness ratio of a compression member is defined as the ratio of its effective length to the least radius of gyration.
Modes of Failure of Columns
The mode of failure of a column depends upon the various parameters. These parameters consist of the slenderness ratio, effective length, etc.
Rankine’s Formula
Rankine proposed an empirical formula for columns ranging from very short to long struts. He proposed the relation.
1/ PR =1 / Pc + 1 / Pe
Pc = σC. A = ultimate load for a strut
Eulerian crippling load for the standard case;



